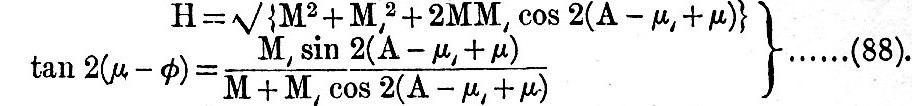V. SYNTHETIC METHOD (cont.)

31. Synthesis of Lunar and Solar Semi-Diurnal Tides

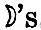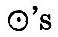Let A be the excess of over R.A., so that

The synthesis is then completed by writing

Then H is the height of the total semi-diurnal tide and φ/(γ - dα/dt) or φ/γ - σ) or 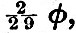, when φ is given in degrees, is the "interval" from the moon’s transit to high water.

The formulae for H and φ may be written

They may be reduced to a form adapted for logarithmic calculation. Since A goes through its period in a lunation, it follows that H and φ have inequalities with a period of half a lunation. These are called the "fortnightly or semi-menstrual inequalities" in the height and interval.

Spring tide obviously occurs when Since the mean value of A is s - h (the difference of the mean longitudes), and since the mean values of μ and are , it follows that the mean value of the period elapsing after full moon and change of moon up to spring tide is (κ₈ - κ_m/2(σ - η). The association of spring tide with full and change is obvious, and a fiction has been adopted by which it is held that spring tide is generated in those configurations of the moon and sun, but takes some time to reach the port of observation. Accordingly (κ₈ - κ_m/2(σ - η) has been called the "age of the tide." The average age is about 36 hours as far as in observations have yet been made. The age of the tide appears not in general to differ very much from the ages of the declinational and parallactic inequalities.

In computing a tide-table it is found practically convenient not to use A, which is the difference of R.A.’s at the unknown time of high water, but to refer the tide to A₀, the difference of R.A.’s at the time of the moon’s transit. It is clear that A₀ is the apparent time of the moon’s transit reduced to angle at 15º per hour. We have already remarked that φ/(γ - dα/dt) is the interval from transit to high water, and hence at high water

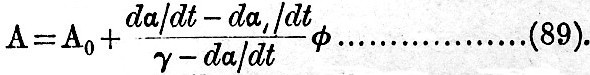
As an approximation we may attribute, to all the quantities in the second term their mean values, and we then have

This approximate formula (90) may be used in computing from (88) the fortnightly inequality in the "height" and "interval."

In this investigation we have supposed that the declinational and parallactic corrections are applied to the lunar and solar tides before their synthesis; but it is obvious that the process might be reversed, and that we may form a table of the fortnightly inequality based on mean values H_m and H_s, and afterwards apply corrections. This is the process usually adopted, but it is less exact. The labour of computing the fortnightly inequality, especially by graphical methods, is not great, and the plan here suggested seems preferable.






Read the rest of this article:
Tides - Table of Contents