
After this brief resume we may once again turn our thoughts to the main results of our researches as summed up on the Dodecahedron within the perfect Sphere.
It would almost seem that our original Qabalistic conceptions have led us out of the realm of thought usually linked with the Hebrew Qabalah into an atmosphere of Intelligibles which is associated with the philosophy of the Masters in ancient Greece. Or, we may say, as our conceptions have expanded towards the Universal, we have contacted another set of teachings, thus uniting Hebrew and Greek thought. Plato informs us in his Republic: "Geometry rightly treated is the knowledge of the Eternal," and he is reported by Plutarch to have said that "God is always geometrizing." Nor was the conception of the Universe in the form of the Dodecahedron unknown to Plato, for in his Timaeus this idea is clearly indicated.
But just how this solid figure was built up so as to symbolize the Universe in all its details, if known to the ancients, was not revealed. Let us see, however, what hints are to be found in the writings of other authorities.
Proclus, in his Introduction to Books II and III of Plato's Republic, says: "But the former (Vulcan) artificially fabricated the whole sensible order, and filling it with physical reasons and powers. He also fashioned twenty tripods about the heavens, that he may adorn them with the most perfect of the many sided figures and fabricates various and many-formed sublunary species." To which Thomas Taylor, the great Cambridge Platonist, adds, "Viz.: the dodecahedron, which is bounded by twelve equal and equilateral pentagons, and consists of twenty solid angles, of which the tripods of Vulcan are images; for every angle of the dodecahedron is formed from the junction of three lines."
There are several references to the dodecahedron in Madam Blavatsky's Secret Doctrine, among which the following is of special interest. "The most distinct and the one prevailing idea, found in all ancient teaching, with reference to Cosmic Evolution and the first 'creation' of our Globe with all its products, organic and inorganic-strange word for an Occultist to use! -- is that the whole Kosmos has sprung from the Divine Thought. This Thought impregnates Matter, which is co-eternal with the One Reality; and all that lives and breathes evolves from the Emanations of the One Immutable Parabrahman-Mulaprakriti, the Eternal One-Root. The former of these, in its aspect of the Central Point turned inward, so to say, into regions quite inaccessible to human intellect is Absolute Abstraction; whereas, in its aspect as Mulaprakriti the Eternal Root of All, it gives one at least some hazy comprehension of the Mystery of Being.
"Therefore, it was taught in the inner temples that the visible Universe of Spirit and Matter is but the Concrete Image of the Ideal Abstraction; it was built on the Model of the First Divine Idea. Thus, our Universe existed from eternity in a latent state. The Soul animating this purely Spiritual Universe is the Central Sun, the highest Deity Itself. It was not the One who built the concrete form of the idea, but the First Begotten; and, as it was constructed on the geometrical figure of the dodecahedron the First Begotten 'was pleased to employ 12,000 years in its creation.' "
It would seem, from the above, that the "Model of the First Divine Idea" may have been very much in harmony with the ideal Formative Principle we have been studying.
This conception of the Universe as a Dodecahedron appears if not to have originated with Plato, to have first been mentioned by him in his writings. All the references I have so far discovered in connection with the idea, can be traced back to this source. Mr. Harley Burr Alexander, of the University of Nebraska, makes some interesting remarks in this connection (Nature and Human Nature, P. 378). " 'We must conceive,' says Plato, 'of three natures: first, that which is in process of generation, and this would be the world of nature as we experience it; second, that in which the generation takes place, and this is the recipient or matrix of nature; and third, that of which the generated world is an image, and this is the cosmic reason or form. We may liken the receiving principle to a mother, and the source or spring to a father, and the intermediate nature to a child,' he says, and we think immediately of the mythopoetic union of Earth and Heaven and the Life of Nature which is its offspring. But for Plato this is a mere trope; he does not rest without being scientifically explicit. 'There are three kinds of being: that which is uncreated and indestructible, changeless, eternal, imperceptible to any sense, open only to the contemplation of the intelligence, and this is the principle of the Father, the ideal or formal essence of the world; again, that which is sensible and created and always in motion, the Child, the world of change and life; and finally, there is a third nature, the Mother, which, like the Father, is eternal and admits not of destruction, which provides a home for all created things, and is apprehended 'without the help of sense, by a kind of spurious reason, and is indeed hardly real.' This nature is space, and we beholding as in a dream, say of all existence that it must of necessity be in some place and occupy a space, but that what is neither in heaven nor in earth has no existence.
"This mothering space which is hardly real, yet is the cause of the determinism of nature, Plato identifies as the material element of being. As pure matter, it is purely indeterminate, but it is receptive of all determinations. The four elements, earth, air, fire and water, are formed from it, for 'the mother substance becomes earth and air, insofar as she receives the impressions of them.' Plato's conception of the formation of these elements from the original substance was as purely mathematical as are our modern physical notions. 'God fashioned them by form and number,' he says: and the forms which he assigned were the forms of the regular solids. Thus the form of the fiery element is the pyramid, of air, the octahedron; of water, the icosahedron; of earth, the cube. The fifth solid, the Dodecahedron, is the form of the universe as a whole, or perhaps one might say the scaffold upon which the spherical universe is constructed. Further, these elements are themselves compounded of simpler mathematical forms, the pyramid, octahedron and icosahedron of equilateral, the cube of isosceles triangles; so that if we regard the elements as molecules, we may view the triangles as atoms of the material substrate.
"Doubtless it was this geometrical account of matter which gave rise to the saying ascribed to Plato that 'God always geometrizes'-for God, says Plutarch in his commentary on the saying, made the world in no other way than by setting terms to infinite and chaotic matter."
There seems to have been many attempts to find a solution to this problem raised by Plato; and apparently as many failures. For instance, the author of "The Canon" remarks; "Nearly all the old philosophers devised an harmonic theory with respect to the universe, and the practice continued till the old mode of philosophizing died out.
"Kepler, in order to demonstrate the Platonic doctrine, that the universe was formed of the five regular solids, proposed the following rule. 'The earth is a circle, the measurer of all. Round it describe a dodecahedron; the circle inclosing this will be Mars. Round Mars describe a tetrahedron; the sphere inclosing this will be Jupiter. Describe a cube round Jupiter; the sphere containing this will be Saturn. Now inscribe in the earth an icosahedron; the circle inscribed in it will be Venus. Inscribe an octahedron in Venus; the circle inscribed in it will be Mercury.' ("Mysterium Cosmographicum," 1596).
"This rule cannot be taken seriously as a real statement of the proportions of the cosmos, for it bears no resemblance to the ratios published by Copernicus in the beginning of the sixteenth century. Yet Kepler was very proud of his formula, and said he valued it more than the Electorate of Saxony. It was also approved by those two eminent authorities, Tycho and Galileo, who evidently understood it. Kepler himself never gives the least hint of how his precious rule is to be interpreted."
The author of "The Canon" then submits a proposed plan of finding universal measurements symbolically concealed in the above rule, but in order to do this he assumes that the figures need not be taken as solids but as so many regular plane polygons. But this seems to me quite a departure from the problem. Also Plato connected these solids with the Elements rather than with the Planets.
But I think our complex solid will be found to contain those mentioned by Plato, although such a thought did not enter my mind until quite lately.
It should be remembered that the Qabalists attribute the four elements to Malkuth, which is often called the Sphere of the Elements. Now Malkuth has remained a perfect Sphere in our plan and in fact represents the Material Substance of the Universe. But the Qabalists have in particular attributed to Malkuth the Element of Earth, while to the next three Sephiroth, Yesod, Hod, and Netzach, have been assigned Air, Water, and Fire.
Earth, as Matter, has always been symbolized by the Cube, or the Cube within the Sphere, and we may well consider this Cube to be concealed in the Sphere of Malkuth. The lower section of the Prismatic Tree, that representing the four lower Sephiroth of the Elements, is in the form of a perfect tetrahedron, that is to say, a solid bounded by four plane triangular faces, each of which is equilateral. And again we find this same solid with a central core of Fire-the Path of Shin-comprising the combinations of Chokmah, Binah, and Tiphereth. Plato particularly attributes this form to Fire.
That portion of our Complete Complex Solid, representing the Elemental Sephiroth; viz., up to the Planes of "The Hierophant" which are penetrated by the Central Paths of Mem, or Water, is composed of twenty of the above solids so conjoined as to produce a perfect icosahedron, viz.: -- a solid bounded by twenty equilateral triangles. This is particularly attributed to Water in the Platonic scheme.
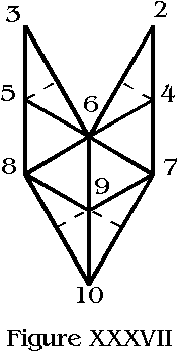
When the progression is made to include Tiphereth, the twenty equilateral planes become points, or tripods which mark out the Dodecahedron, while the Second Progression of the Tree exactly encloses this. The points of the twenty Kethers indicate a similar but larger solid.
Thus we have disposed of four of the five regular solids.
The last, or octahedron, presents some difficulty. This is a solid bounded by eight equal and equilateral plane surfaces, and six summits or vertices. I have so far been unable to discover that such a solid exists within our complex solid while deriving its surfaces and angles from regular sections of the Tree.
But what a first sight appears a difficulty may possibly become a clue when we consider that this form is attributed to the Elements of Air. Fire, Water, and Earth are all perceptible to our sense of sight-not so Air. I do not want this to appear as an evasion of the issue, for in any case there are interesting indications of this missing form in the Tree of Life as originally outlined.
It will be found that four small equilateral triangles are shown on the Tree uniting the Six Sephiroth which are attributed to Vau in the Four-lettered Name, and Vau is the letter of Air. These four triangles are arranged thus:

It will be found that if a plane surface be folded on the lines where these triangles join, and if the points represented by Chesed and Geburah be drawn together, it forms exactly one-half of an Octahedron. And it may further be noted that, leaving out from the figure of the whole Tree that portion which represents our tetrahedron, plus Kether, the remainder has the following form:
This, it will be observed, represents the portion just described which makes up the half-octahedron, together with just enough additional surface material to form four other equilateral triangles required for the completion of the figure. But, without severing the fragments, we cannot take a sheet of paper and fold it into the desired shape, as was the case when making the solid prismatic Tree itself.
But even in the latter case a very interesting thing will be noticed-the summit of the Supernal Triad disappears. For, whether we take Three Trees connected at the side thus:

or Three Trees radiating from Kether thus:
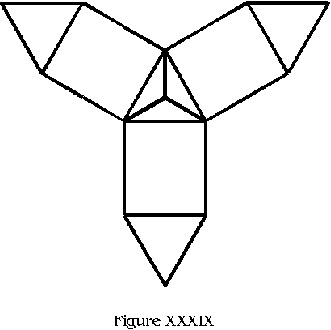
we find, upon experiment in folding, that although the lower triangle fits together perfectly, when we bend the paper back along the lines of the Path of Aquarius we get a flat top with Kether on a level with Chokmah and Binah. Therefore, although for the sake of retaining the appearance of the original two-dimensional figure, we must build up a Summit to represent Kether; we do so, as it were, with apologies to the Supreme One which must ever remain unmanifest to our lower senses. In other words the Summit of the Supernals cannot be conceived as a "solid," or even as partaking of "form" in the realm of ideas. This, rather than detracting from our plan, leads us to a most important truth, viz.: that in our researches we must never for a moment forget the Superessential excellence of the ONE and the GOOD. For, as Simplicius beautifully observes, "It is requisite that he who ascends to the principle of things, should investigate whether it is possible there can be anything better than the supposed principle; and if something more excellent is found, the same inquiry should again be made respecting that, till we arrive at the highest conception, than which we have no longer any more venerable. Nor should we stop in our ascent till we find this to be the case. For there is no occasion to fear that our progression will be through an unsubstantial void, by conceiving something about the first principles which is greater than and surpasses their nature. For it is not possible for our conceptions to take such a mighty leap as to equal, and much less to pass beyond the dignity of the first principle of things." He adds: "This, therefore, is one and the best extension (of the soul) to (the highest) God, and is as much as possible irreprehensible; viz., to know firmly, that by ascribing to him the most venerable excellencies we can conceive, and the most holy and primary names and things, we ascribe nothing to him which is suitable to his dignity. It is sufficient, however, to procure our pardon (for the attempt) that we can attribute to him nothing superior."
And in respect of our pardon we may devoutly hope that Simplicius was right.